最近總結了一些數據結構和算法相關的題目,這是第一篇文章,關于二叉樹的。先上二叉樹的數據結構:
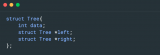
class TreeNode{ int val; //左孩子 TreeNode left; //右孩子 TreeNode right;}
二叉樹的題目普遍可以用遞歸和迭代的方式來解
1. 求二叉樹的最大深度
int maxDeath(TreeNode node){ if(node==null){ return 0; } int left = maxDeath(node.left); int right = maxDeath(node.right); return Math.max(left,right) + 1;}
2. 求二叉樹的最小深度
int getMinDepth(TreeNode root){ if(root == null){ return 0; } return getMin(root); } int getMin(TreeNode root){ if(root == null){ return Integer.MAX_VALUE; } if(root.left == null&&root.right == null){ return 1; } return Math.min(getMin(root.left),getMin(root.right)) + 1; }
3. 求二叉樹中節點的個數
int numOfTreeNode(TreeNode root){ if(root == null){ return 0; } int left = numOfTreeNode(root.left); int right = numOfTreeNode(root.right); return left + right + 1; }
4. 求二叉樹中葉子節點的個數
int numsOfNoChildNode(TreeNode root){ if(root == null){ return 0; } if(root.left==null&&root.right==null){ return 1; } return numsOfNodeTreeNode(root.left)+numsOfNodeTreeNode(root.right); }
5. 求二叉樹中第k層節點的個數
int numsOfkLevelTreeNode(TreeNode root,int k){ if(root == null||k<1){ ? ? ? ? ? ? ? ?return 0; ? ? ? ? ? ?} ? ? ? ? ? ?if(k==1){ ? ? ? ? ? ? ? ?return 1; ? ? ? ? ? ?} ? ? ? ? ? ?int numsLeft = numsOfkLevelTreeNode(root.left,k-1); ? ? ? ? ? ?int numsRight = numsOfkLevelTreeNode(root.right,k-1); ? ? ? ? ? ?return numsLeft + numsRight; ? ? ? ?}
6. 判斷二叉樹是否是平衡二叉樹
boolean isBalanced(TreeNode node){ return maxDeath2(node)!=-1; } int maxDeath2(TreeNode node){ if(node == null){ return 0; } int left = maxDeath2(node.left); int right = maxDeath2(node.right); if(left==-1||right==-1||Math.abs(left-right)>1){ return -1; } return Math.max(left, right) + 1; }
7.判斷二叉樹是否是完全二叉樹
什么是完全二叉樹呢?參見
boolean isCompleteTreeNode(TreeNode root){ if(root == null){ return false; } Queue
8. 兩個二叉樹是否完全相同
boolean isSameTreeNode(TreeNode t1,TreeNode t2){ if(t1==null&&t2==null){ return true; } else if(t1==null||t2==null){ return false; } if(t1.val != t2.val){ return false; } boolean left = isSameTreeNode(t1.left,t2.left); boolean right = isSameTreeNode(t1.right,t2.right); return left&&right; }
9. 兩個二叉樹是否互為鏡像
boolean isMirror(TreeNode t1,TreeNode t2){ if(t1==null&&t2==null){ return true; } if(t1==null||t2==null){ return false; } if(t1.val != t2.val){ return false; } return isMirror(t1.left,t2.right)&&isMirror(t1.right,t2.left); }
10. 翻轉二叉樹or鏡像二叉樹
TreeNode mirrorTreeNode(TreeNode root){ if(root == null){ return null; } TreeNode left = mirrorTreeNode(root.left); TreeNode right = mirrorTreeNode(root.right); root.left = right; root.right = left; return root; }
11. 求兩個二叉樹的最低公共祖先節點
TreeNode getLastCommonParent(TreeNode root,TreeNode t1,TreeNode t2){ if(findNode(root.left,t1)){ if(findNode(root.right,t2)){ return root; }else{ return getLastCommonParent(root.left,t1,t2); } }else{ if(findNode(root.left,t2)){ return root; }else{ return getLastCommonParent(root.right,t1,t2) } } } // 查找節點node是否在當前 二叉樹中 boolean findNode(TreeNode root,TreeNode node){ if(root == null || node == null){ return false; } if(root == node){ return true; } boolean found = findNode(root.left,node); if(!found){ found = findNode(root.right,node); } return found; }
12. 二叉樹的前序遍歷
迭代解法
ArrayList
遞歸解法
ArrayList
13. 二叉樹的中序遍歷
ArrayList
14.二叉樹的后序遍歷
ArrayList
15.前序遍歷和后序遍歷構造二叉樹
TreeNode buildTreeNode(int[] preorder,int[] inorder){ if(preorder.length!=inorder.length){ return null; } return myBuildTree(inorder,0,inorder.length-1,preorder,0,preorder.length-1); } TreeNode myBuildTree(int[] inorder,int instart,int inend,int[] preorder,int prestart,int preend){ if(instart>inend){ return null; } TreeNode root = new TreeNode(preorder[prestart]); int position = findPosition(inorder,instart,inend,preorder[start]); root.left = myBuildTree(inorder,instart,position-1,preorder,prestart+1,prestart+position-instart); root.right = myBuildTree(inorder,position+1,inend,preorder,position-inend+preend+1,preend); return root; } int findPosition(int[] arr,int start,int end,int key){ int i; for(i = start;i<=end;i++){ ? ? ? ? ? ?if(arr[i] == key){ ? ? ? ? ? ? ? ?return i; ? ? ? ? ? ?} ? ? ? ?} ? ? ? ?return -1; ? ?}
16.在二叉樹中插入節點
TreeNode insertNode(TreeNode root,TreeNode node){ if(root == node){ return node; } TreeNode tmp = new TreeNode(); tmp = root; TreeNode last = null; while(tmp!=null){ last = tmp; if(tmp.val>node.val){ tmp = tmp.left; }else{ tmp = tmp.right; } } if(last!=null){ if(last.val>node.val){ last.left = node; }else{ last.right = node; } } return root; }
17.輸入一個二叉樹和一個整數,打印出二叉樹中節點值的和等于輸入整數所有的路徑
void findPath(TreeNode r,int i){ if(root == null){ return; } Stack
18.二叉樹的搜索區間
給定兩個值 k1 和 k2(k1 < k2)和一個二叉查找樹的根節點。找到樹中所有值在 k1 到 k2 范圍內的節點。即打印所有x (k1 <= x <= k2) 其中 x 是二叉查找樹的中的節點值。返回所有升序的節點值。
ArrayList
19.二叉樹的層次遍歷
ArrayList
20.二叉樹內兩個節點的最長距離
二叉樹中兩個節點的最長距離可能有三種情況:1.左子樹的最大深度+右子樹的最大深度為二叉樹的最長距離2.左子樹中的最長距離即為二叉樹的最長距離3.右子樹種的最長距離即為二叉樹的最長距離因此,遞歸求解即可
private static class Result{ int maxDistance; int maxDepth; public Result() { } public Result(int maxDistance, int maxDepth) { this.maxDistance = maxDistance; this.maxDepth = maxDepth; } } int getMaxDistance(TreeNode root){ return getMaxDistanceResult(root).maxDistance; } Result getMaxDistanceResult(TreeNode root){ if(root == null){ Result empty = new Result(0,-1); return empty; } Result lmd = getMaxDistanceResult(root.left); Result rmd = getMaxDistanceResult(root.right); Result result = new Result(); result.maxDepth = Math.max(lmd.maxDepth,rmd.maxDepth) + 1; result.maxDistance = Math.max(lmd.maxDepth + rmd.maxDepth,Math.max(lmd.maxDistance,rmd.maxDistance)); return result; }
21.不同的二叉樹
給出 n,問由 1…n 為節點組成的不同的二叉查找樹有多少種?
int numTrees(int n ){ int[] counts = new int[n+2]; counts[0] = 1; counts[1] = 1; for(int i = 2;i<=n;i++){ ? ? ? ? ? ?for(int j = 0;j
22.判斷二叉樹是否是合法的二叉查找樹(BST)
一棵BST定義為:節點的左子樹中的值要嚴格小于該節點的值。節點的右子樹中的值要嚴格大于該節點的值。左右子樹也必須是二叉查找樹。一個節點的樹也是二叉查找樹。
public int lastVal = Integer.MAX_VALUE; public boolean firstNode = true; public boolean isValidBST(TreeNode root) { // write your code here if(root==null){ return true; } if(!isValidBST(root.left)){ return false; } if(!firstNode&&lastVal >= root.val){ return false; } firstNode = false; lastVal = root.val; if (!isValidBST(root.right)) { return false; } return true; }
深刻的理解這些題的解法思路,在面試中的二叉樹題目就應該沒有什么問題
-
二叉樹
+關注
關注
0文章
74瀏覽量
12629
原文標題:一篇文章搞定面試中的二叉樹
文章出處:【微信號:TheAlgorithm,微信公眾號:算法與數據結構】歡迎添加關注!文章轉載請注明出處。
發布評論請先 登錄
二叉查找樹(GIF動圖講解)
二叉樹層次遍歷算法的驗證
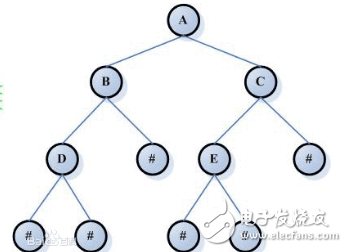





 關于二叉樹一些數據結構和算法相關的題目
關于二叉樹一些數據結構和算法相關的題目











評論