在檢測物體的輪廓時，我們通常會使用到opencv中的findcontour和drawcontour，比較常用而且效果不錯。那么findcontour是基于什么原理來實現輪廓的提取呢？

　　輪廓的提取與描述

　　在目標識別中我們首先要把感興趣的目標提取出來，而一般常見的步驟都是通過顏色或紋理提取出目標的前景圖（一幅黑白圖像，目標以白色顯示在圖像中），接下來我們要對前景圖進行分析進一步地把目標提取出來，而這里常常用到的就是提取目標的輪廓。

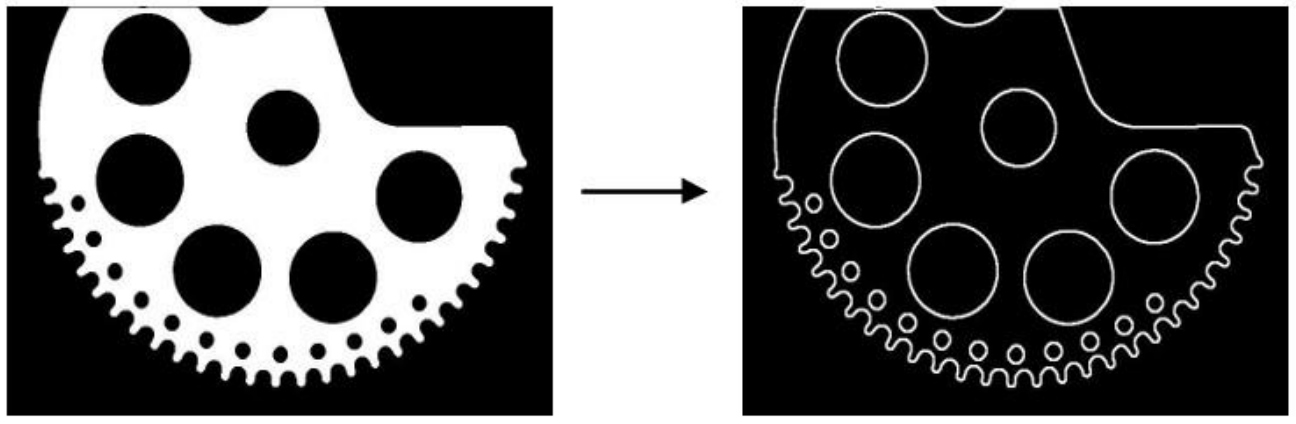
　　OpenCV里提取目標輪廓的函數是findContours，它的輸入圖像是一幅二值圖像，輸出的是每一個連通區域的輪廓點的集合：vector《vector《Point》》。外層vector的size代表了圖像中輪廓的個數，里面vector的size代表了輪廓上點的個數。下面我們通過實例來看函數的用法。

　　view plain copy print？

　　int main（）

　　{

　　using namespace cv;

　　Mat image=imread（“。。/shape.png”）;

　　cvtColor（image，image，CV_BGR2GRAY）;

　　vector《vector《Point》》 contours;

　　// find

　　findContours（image，contours，CV_RETR_EXTERNAL，CV_CHAIN_APPROX_NONE）;

　　// draw

　　Mat result（image.size（），CV_8U，Scalar（0））;

　　drawContours（result，contours，-1，Scalar（255），2）;

　　namedWindow（“contours”）;

　　imshow（“contours”，result）;

　　waitKey（）;

　　return 0;

　　}

　　int main（）

　　{

　　using namespace cv;

　　Mat image=imread（“。。/shape.png”）;

　　cvtColor（image，image，CV_BGR2GRAY）;

　　vector《vector《Point》》 contours;

　　// find

　　findContours（image，contours，CV_RETR_EXTERNAL，CV_CHAIN_APPROX_NONE）;

　　// draw

　　Mat result（image.size（），CV_8U，Scalar（0））;

　　drawContours（result，contours，-1，Scalar（255），2）;

　　namedWindow（“contours”）;

　　imshow（“contours”，result）;

　　waitKey（）;

　　return 0;

　　}

　　上面程序中包含了2個函數，第一個是查找輪廓函數，它的第三個參數說明查找輪廓的類型，這里我們使用的是外輪廓，還可以查找所有輪廓，即

　　包括一些孔洞的部分，像圖像人物胳膊與腰間形成的輪廓。第4個參數說明了輪廓表示的方法，程序中的參數說明輪廓包括了所有點，也可以用其

　　他參數讓有點直線的地方，只保存直線起始與終點的位置點，具體參數用法可以參考手冊里函數的介紹。

　　第二個函數drawContours是一個畫輪廓的函數，它的第3個參數程序里設置-1表示所有的輪廓都畫，你也可以指定要畫的輪廓的序號。

　　提取到輪廓后，其實我們更關心的是如果把這些輪廓轉換為可以利用的特征，也就是涉及到輪廓的描述問題，這時就有多種方法可以選擇，比如矢

　　量化為多邊形、矩形、橢圓等。OpenCV里提供了一些這樣的函數。

　　［cpp］ view plain copy print？

　　// 輪廓表示為一個矩形

　　Rect r = boundingRect（Mat（contours［0］））;

　　rectangle（result， r， Scalar（255）， 2）;

　　// 輪廓表示為一個圓

　　float radius;

　　Point2f center;

　　minEnclosingCircle（Mat（contours［1］）， center， radius）;

　　circle（result， Point（center）， static_cast《int》（radius）， Scalar（255）， 2）;

　　// 輪廓表示為一個多邊形

　　vector《Point》 poly;

　　approxPolyDP（Mat（contours［2］）， poly， 5， true）;

　　vector《Point》：：const_iterator itp = poly.begin（）;

　　while （itp ！= （poly.end（） - 1））

　　{

　　line（result， *itp， *（itp + 1）， Scalar（255）， 2）;

　　++itp;

　　}

　　line（result， *itp， *（poly.begin（））， Scalar（255）， 2）;

　　// 輪廓表示為凸多邊形

　　vector《Point》 hull;

　　convexHull（Mat（contours［3］）， hull）;

　　vector《Point》：：const_iterator ith = hull.begin（）;

　　while （ith ！= （hull.end（） - 1））

　　{

　　line（result， *ith， *（ith + 1）， Scalar（255）， 2）;

　　++ith;

　　}

　　line（result， *ith， *（hull.begin（））， Scalar（255）， 2）;

　　// 輪廓表示為一個矩形

　　Rect r = boundingRect（Mat（contours［0］））;

　　rectangle（result， r， Scalar（255）， 2）;

　　// 輪廓表示為一個圓

　　float radius;

　　Point2f center;

　　minEnclosingCircle（Mat（contours［1］）， center， radius）;

　　circle（result， Point（center）， static_cast《int》（radius）， Scalar（255）， 2）;

　　// 輪廓表示為一個多邊形

　　vector《Point》 poly;

　　approxPolyDP（Mat（contours［2］）， poly， 5， true）;

　　vector《Point》：：const_iterator itp = poly.begin（）;

　　while （itp ！= （poly.end（） - 1））

　　{

　　line（result， *itp， *（itp + 1）， Scalar（255）， 2）;

　　++itp;

　　}

　　line（result， *itp， *（poly.begin（））， Scalar（255）， 2）;

　　// 輪廓表示為凸多邊形

　　vector《Point》 hull;

　　convexHull（Mat（contours［3］）， hull）;

　　vector《Point》：：const_iterator ith = hull.begin（）;

　　while （ith ！= （hull.end（） - 1））

　　{

　　line（result， *ith， *（ith + 1）， Scalar（255）， 2）;

　　++ith;

　　}

　　line（result， *ith， *（hull.begin（））， Scalar（255）， 2）;

　　程序中我們依次畫了矩形、圓、多邊形和凸多邊形。最終效果如下：

　　對連通區域的分析到此遠遠沒有結束，我們可以進一步計算每一個連通區域的其他屬性，比如：重心、中心矩等特征，這些內容以后有機會展開來寫。

　　以下幾個函數可以嘗試：minAreaRect：計算一個最小面積的外接矩形，contourArea可以計算輪廓內連通區域的面積；pointPolygenTest可以

　　用來判斷一個點是否在一個多邊形內。mathShapes可以比較兩個形狀的相似性，相當有用的一個函數。